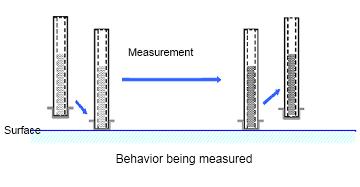
What is Contact Angle?
Sessile Drop – Static Contact Angle
Suppose a liquid droplet is deposited onto a solid surface. In that case, it will form a contact angle depending on its surface tension, the surface tension of the solid substrate, and the interfacial tension between solid and liquid.
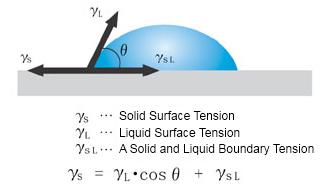
This equation is referred to as the "Young equation", and the angle θ formed by the solid surface and the tangent of the droplet is called the "contact angle".
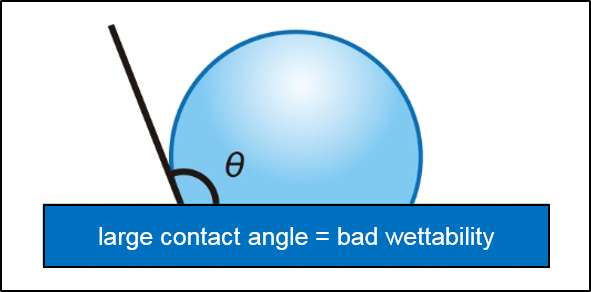
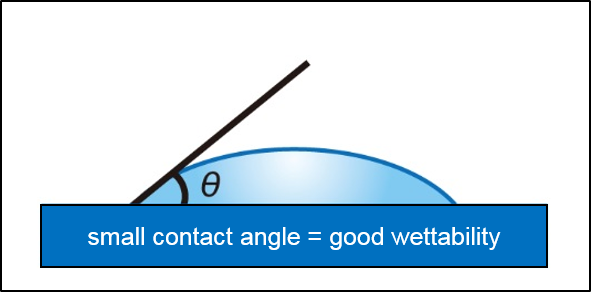
The "contact angle" is intuitive and easy to understand as an indicator of wettability and has been adopted widely in industrial fields as an evaluation method of surfaces.
Sessile Drop – Function of Time
The contact angle measurement in the preceding paragraph is performed continuously to observe variations over time. Although there is no precise definition between dynamic and static, we treat it as one type of dynamic contact angle for one second or fewer intervals. This can also be used to follow absorption and other volatile situations.
Captive Bubble
Contact angle measurements typically measure the wettability of a solid by a liquid in a gaseous phase. Using a special kit, contact angle measurements of a liquid or an air bubble are possible in a surrounding liquid bulk phase.
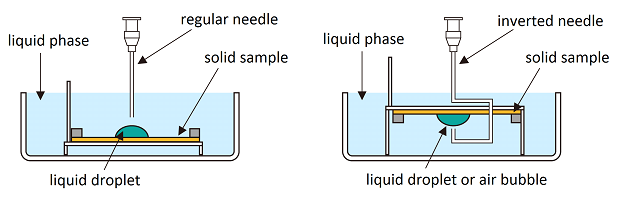
The left image illustrates the measurement of a liquid droplet with a higher density than the bulk liquid phase. In contrast, the right image shows an air bubble or a liquid droplet with a lower density than the bulk phase. Here, the air bubble or droplet is deposited by an inverted needle from beneath the solid sample.
Contact Angle Analysis Methods
θ/2 method
The θ/2 or height-width method is generally used to determine the contact angle. The equation given below determines the contact angle. Where radius r and height h of the droplet.
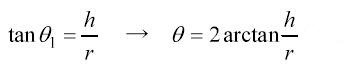
In addition, the contact angle is obtained from the angle of straight lines connecting the left and right ends and the apex of the droplet against a solid surface.
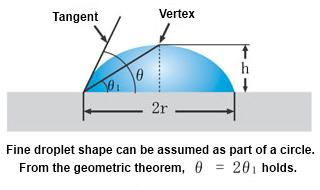
In the θ/2 method, since the shape of the droplet is assumed to be part of the outline of an imaginary circle, measurement is done with a tiny droplet that can ignore the effect of gravitational force.
Tangent method
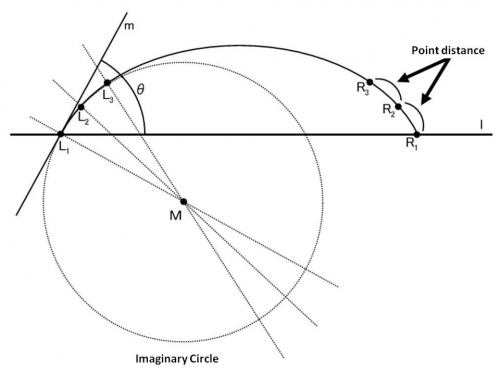
The droplet's shape is assumed to be part of the outline of an imaginary circle. This method obtains the center of the imaginary circle and determines the contact angle as the angle and straight lines tangent to the circle. For example, from the figure below, the three arc points L1, L2, and L3 form the imaginary circle. The angle between the tangent line m and the drop baseline l is the left contact angle. The right contact angle can be measured similarly using points R1, R2, and R3.
The contact angle is determined as the average of the left and right endpoints. Conversely, the Tangent method allows one to independently determine the contact angle by the left and right endpoints. Thus, it is an effective measurement method for uneven surfaces with different left and right contact angles.
Curve fitting method
Assuming that the droplet's contour shape forms part of a true circle or ellipse, the Least square method is performed using the coordinates of all observations (fitting section) in a given interval. This calculation determines the perfect circle or ellipse parameters, and the contact angle differential coefficients of the endpoint are calculated.
With the Tangent method, a perfect circle has been assumed as the contour shape. However, compared with the results of fitting the perfect circle, the second is the better-fitting perfect circle as it uses more coordinates for a smaller variation.
On the assumption that the droplet is at rest on a solid surface and reaches equilibrium quickly if compared to differences at a specific time after the droplet deposition, the contact angle data of the preceding paragraph is beneficial. However, this only applies if the data is not altered much. For example, assuming a case of various states, such as coating or cleaning, where the liquid and solid interface is dynamic, sufficient data is not obtained.
This case simulates (with the advancing and receding contact angle) a dynamic situation where the interface of the liquid droplet moves and has been increasing.
Extension/contraction (captive drop) method
The contact angle of a droplet on a solid surface changes when a needle tip is stuck in the droplet and the volume is controlled through the needle. This method measures the dynamic hysteresis of the contact angle by increasing or decreasing the droplet volume. The contact angle created during the volume increase is defined as the advancing angle and the receding angle while decreasing it.
Advancing angle by increasing the sessile drop volume
Receding angle by decreasing the sessile drop volume
Compared to measuring the static contact angle, the advancing and receding angles deliver more vital information in evaluating dynamic wetting in coating processes such as spin-coating and roll-coating, as they are closer to real coating processes.
Sliding method – measurement of roll-off angles
When a droplet is deposited on a flat solid surface, and the surface is tilted, it will start to roll or slide off at a certain degree. This angle is defined as the sliding or roll-off angle α.
The contact angle at the lower end is the advancing angle θa, and the one on the upper end is the receding angle θr.
 θa: Advancing angle
θa: Advancing angleθr: Receding angle
α: Sliding angle
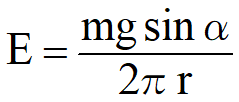 E: Adhesive energy
E: Adhesive energyr: Radius of the droplet
m: Mass of the droplet
g: Acceleration of gravity
α: Sliding angle
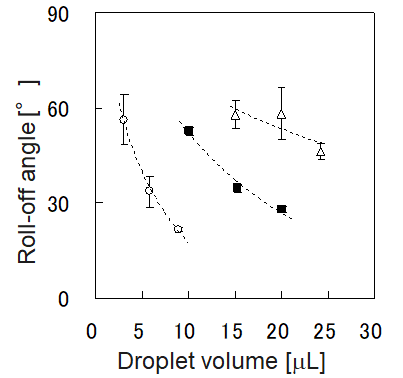
The roll-off angle of a liquid largely depends on its droplet volume, making it impossible to compare the roll-off angles of liquids with different volumes. Here, the adhesive energy between the liquid and the solid's surface at the time when the roll-off angle has been determined can help, as the adhesive energy is not likely to depend on the droplet volume, as the graphs below show.

Related Products
Wilhelmy method
When the measuring unit (Wilhelmy plate) makes contact with the surface of the liquid, the liquid will wet the Wilhelmy plate upwards. In this case, the surface tension acts along the perimeter of the plate and the liquid pulls in the plate. This method detects the pulling force is read and determines the surface tension. At this time, the surface tension can be determined by using a plate material that has a contact angle of 0º with the liquid to the measuring unit (plate). Platinum is commonly used as the plate material.
Conversely, with the Wilhelmy method contact angle measurement, we measured the force of the solid sample when the liquid sample is brought into contact with the suspended sample. The contact angle of the liquid sample and the solid sample is mediated by the difference between the surface tension measurements, and the pulling force is less than the surface tension of the liquid. The contact angle is calculated from the relationship between the surface tension and the decline in force.
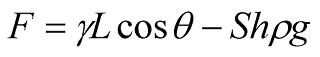
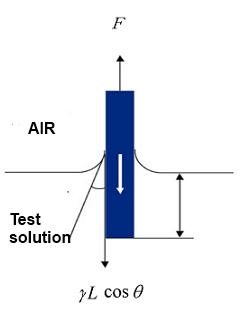
F…Forces acting on the solid (measured)
γ…The surface tension of the liquid (must be known in the case of contact angle measurements)
L…The circumference of the solid sample perimeter (must be known)
θ…The contact angle of the liquid with the solid (assumed to be 0º)
S…The cross-sectional area of the solid sample distance
h…The Immersion depth of the solid sample
ρ…The density of the liquid sample
g…The gravitational acceleration
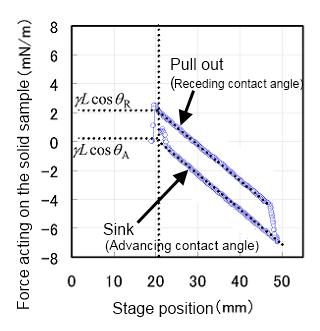
A solid sample is suspended from the balance and immersed in the liquid. Raising the stage will immerse the solid sample deeper into the liquid, and the advancing contact angle θA can be measured. Lowering the stage will pull the solid out of the liquid, and the receding contact angle θR can be measured.
The more the solid sample is immersed in the liquid, the more the buoyant force increases, causing the force acting on the balance to decrease. Therefore, for both the advancing contact angle θA and the receding contact angle θR, the obtained weight curves (see the figure on the right) must be determined through linear regression, and the wetting forces have to be extrapolated to zero-depth immersion.
Related Products
Infiltration Rate method
It is known that the penetration rate of liquid into the powder is represented by the Washburn equation.
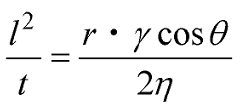
l… Height of the liquid front
t… Time
r… Capillary radius of the compressed powder
γ… Surface tension of the liquid
η… Viscosity of the liquid
θ… Contact angle
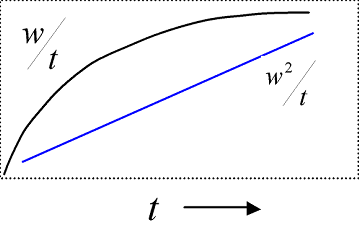
In the actual measurement, a liquid sample is infiltrated into a column filled with powder, and the change in the weight W is tracked for the elapsed time t. Ideally, the linear relationship is obtained by plotting t for W2. The contact angle and infiltration rate are calculated from the slope of this line. In addition, in order to calculate the contact angle, the powder-filled capillary radius value is required besides the values of liquid surface tension and viscosity. That capillary radius value is determined experimentally by measuring the sufficiently wet liquid for the powder and regarding the contact angle as 0º.